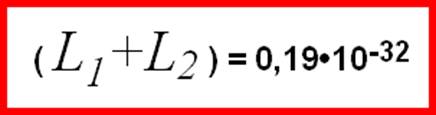|
Назад
Главная страница
Оглавление
Далее
Estimates of the physical properties of a gukuum.
Abstract. The mechanical parameters of gukuum are
calculated: density, elasticity. A comparison with similar
parameters of elastic materials is made.
It remains to find the Lamé coefficients for the gukuum.
It's time to "feel" Gukuum. For this, there are all
possibilities. We consider the most reliable particle: a
neutron. But you can also consider the proton. Earlier, the
inaccuracy of the coefficients of the proton was noted, it
is not symmetric. And the electron is not sufficiently
identified, because there is little experimental data.
We take the previously obtained formulas for the
localized energy.

and
angular momentum of
lok:
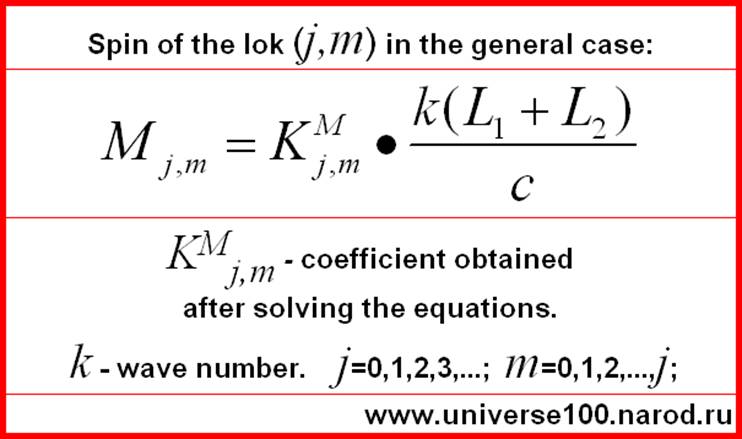
We take
j=1,
m=0
(neutron). In the left part of the equations, we substitute
the real values of the energy
μc2
of the neutron and the spin
ћ/2
of the neutron. We obtain the initial two equations with two
unknowns for the neutron.
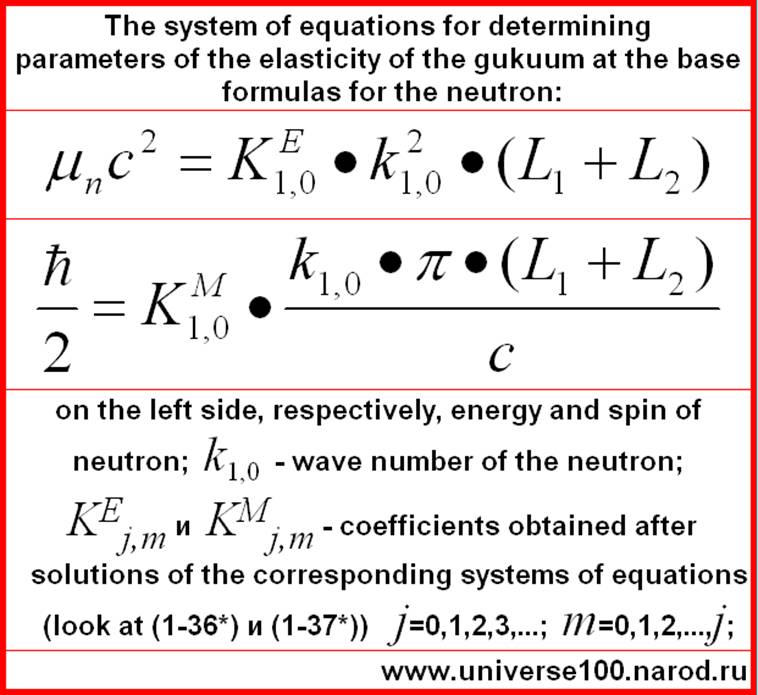
(1-59)
(1-60)
The
solution of these equations is (with allowance for (1-51)):
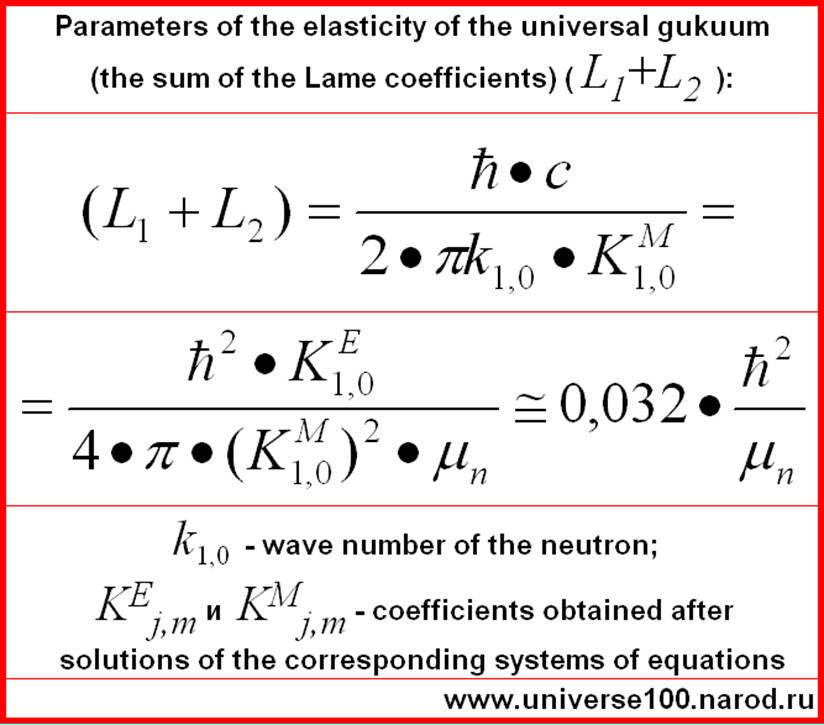
(1-61)
This is how the elastic properties of the Gukuum, the
neutron mass and the Planck constant are related to each
other! Planck's constant determines the elastic properties
of Gukuum (gukuum in traditional physics). And vice versa.
From (1-61) follows a numerical evaluation of the
elastic properties of the Gukuum:
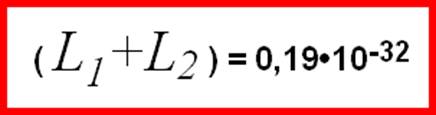
(1-62)
(in the
GHS system).
The following is an estimate of the density of the Gukuum. In accordance
with the laws of elasticity:
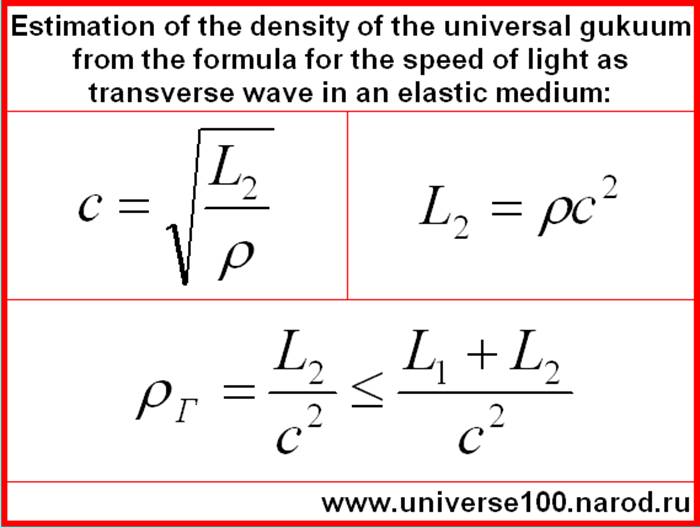
(1-63)
Or in units of the GHS system:
 (1-64)
ρ
is the density of the gukuum. This is only the upper
estimate of the density of the Gukuum, and the lower one can
be equal to zero. Analysis is still being postponed.
As is clear from formulas (1-62) and (1-63), the
elasticity and density of Gukuum prove to be negligible in
comparison with any terrestrial matter. At first glance it
is a paradox. Insignificant in the density and elasticity of
the carrier, but transfers enormous energies. Is it possible
that the elastic parameters of the object formed by the
fluctuation of the Gukuum are tens of orders higher than the
elastic characteristics of the carrier itself? This is
shocking. Compared with terrestrial substances. Here is the
elastic substance: steel. For steel
L1≈L2≈106;
ρsteel≈101;
and
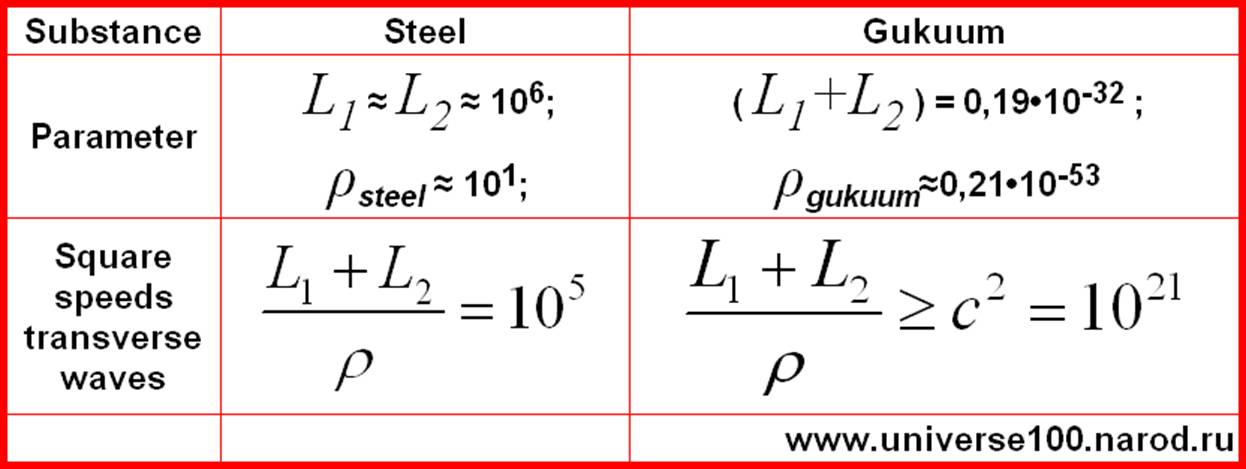
(1-65)
That is due to what the speed of light is many times greater
than the speed of sound in steel. So what is more
"resilient", 1021 or 105
?! That's where it really is. What vortexes of ether can
give the same ratio?
It remains to be seen what each of the two Lame
coefficients for Gukuum is equal to. You can make an
assessment. For steel
L1≈L2.
If this is true for Gukuum, then

(1-66)
(in
the GHS system)
But
why should it be
L1≈L2?
And suddenly
L1>>L2?
That is, Gukuum is easy to shift, but is very heavy on compression? What
if it's a LIQUID? It should be remembered that even if gukuum = liquid,
then we are not talking about motions and vortices of a liquid, but
about elastic waves in a liquid.
But this
assumption does not hold water.
Light on all known experimental results is a transverse wave. This is
the direction of the vectors of the electric and magnetic fields in
electromagnetic waves. And transverse waves are possible only in a
solid. Liquids, gases and ethers allow only longitudinal waves,
compression waves.
It is entirely possible that Gukuum is incompressible, as suggested in
[25]. Formulas still allow this possibility. In this version there are
no estimates for the density of Gukuum.
So, Gukuum was the easiest as an ether. But it is elastic both in
relation to compression, and to a shift (torsion) and capable of
transferring through its oscillations colossal energies. If there was no
elasticity to the shift, there would be no light. There would be no
elementary particles.
I want to note the special moments of the new theory.
First, what was just mentioned: it is possible that the Gukuum is
absolutely incompressible and all objects observed by man are only a
game of stresses. It may well be that our reality is virtual! This is
the first game of zeros - zeros of deformation.
Secondly,
it turns out that this virtuality does not end there. The discovery of
the "winding" law introduces a special functional factor 1/r2
into the integral in the calculation of energy.
Without this factor, the energy integral of the "single loop" does not
converge. That is, how small the constant
Cj
is in solution (1-2), the energy integral is still infinitely large in
amplitude. So this constant is physically zero! That is, a single
localized wave, one of its turns, has a zero amplitude! Another virtual
reality. Another game of zeros! It turns out that everything in the
universe is a game of zeros.
2007.01.05.
Addition. In order to establish the relationship between
L1
and
L2,
it has been suggested that this ratio should somehow correlate with
analogous relations in solid material substances. We have already
mentioned the substance - steel, but I want more persuasiveness. We took
the table of elastic properties from [39]. Something is there, something
we have calculated up to the formulas from the theory of elasticity.
The
elastic modulus
E,
Poisson's ratio σ and the Lame coefficients
L1
and
L2
for various material substances. Based on materials from [39].
In the calculations we used the elasticity formulas:
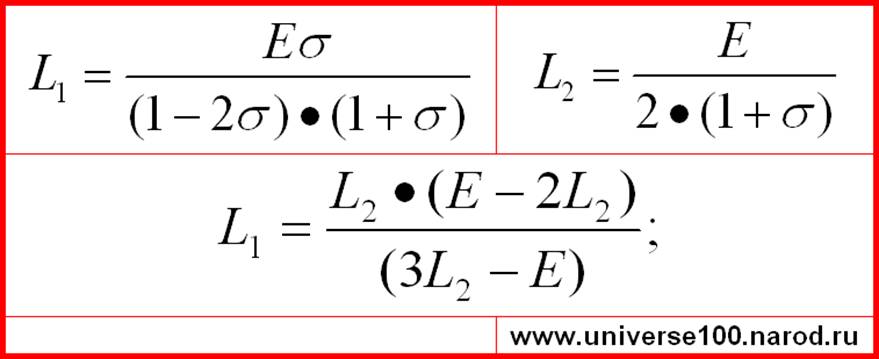
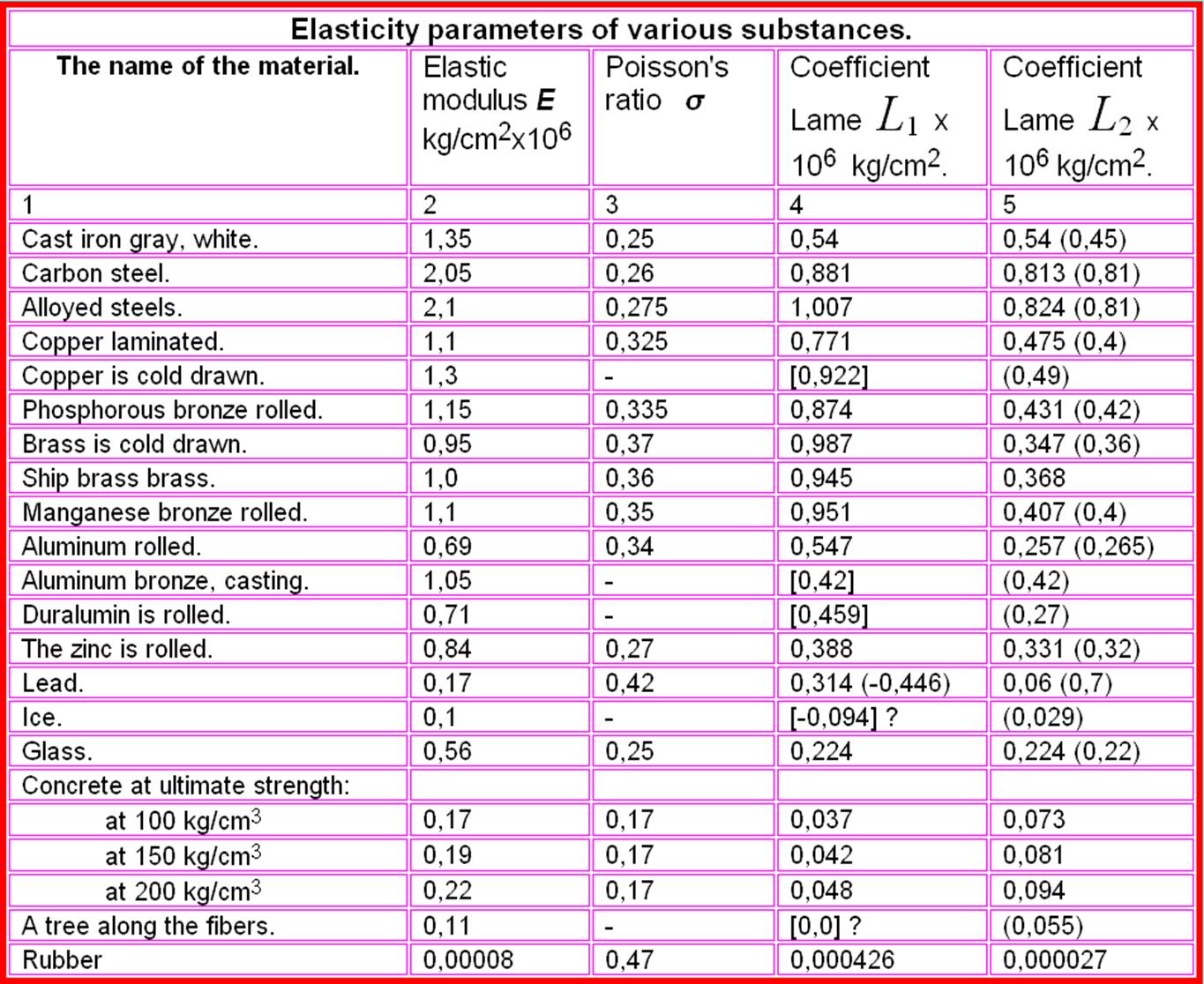
Note.
1) Most of the values in columns 4 and 5 are calculated from the data from
columns 2 and 3. No parentheses are used.
2) The values in the columns in square brackets are calculated based on the
elasticity formulas from the experimental data from the other columns except 2
and 3.
3) In the parentheses - the corresponding experimental data.
Conclusions. 1. The closeness of the values in columns 4 and 5 is seen with
sufficient conviction. This may serve as some confirmation that in Gukuum
L1≈L2.
Provided that the original assumption is correct. For which we can not vouch.
2. There is
an assumption that the universe is arranged in such a way that there are no
fixed numerical constants in the universe. There are only mutual relations
between physical quantities and parameters, expressed through other physical
relationships and parameters. At the heart of everything lies the wave equation
(which is simply an expression of the law of continuity and elasticity of the
continuum of the universe). And the three Lame coefficients of this elasticity
of the Gukuum and all physical quantities (elementary particle masses, fields,
particle charges, Planck's constant, gravitation constant, etc.) are simply
related by certain formulas and have no binding to any absolute numerical
values. In other words, all visible physics and the universe are nothing more
than a game, the interference of infinitesimal perturbations of the continuum.
At the same time, our Universe is unambiguous, and there are no parallel
universes.
Опубликовано: https://www.academia.edu/34568841/Estimates_of_the_physical_properties_of_the_gukuum
Назад
Главная страница
Оглавление
Далее
Страница размещена на
сайте в мае 2005 года |