устройство вселенной, внутреннее устройство элементарных частиц, портреты элементарных частиц, the essence of universe, the nature of matter, the elements of mathematical solutions of wave equation

Назад Главная страница Оглавление Далее

Three classes of localized spherical solutions and one class of cylindrical solutions.

Abstract. The basic solutions of the wave equation in vacuum are obtained, which create the whole variety of matter.

So, again the uniform formula of the universe:

(1-1)

Here, W is the displacement vector of the elastic cosmic gukuum element. c is the speed of light or the speed of transverse waves, determined by the mechanical parameters of the gukuum. Longitudinal waves are not considered.
We start from absolutely reliable results: solutions of the wave equation for displacement, and also physical formulas for an elastic body. The same equation (1-1) expressed in the Cartesian coordinates of the projections of the displacement vector W:

(1-1)

VARIOUS TYPES OF SOLUTIONS of equation (1-1) correspond to different types of oscillatory processes. In particular, a) waves propagating to infinity at the speed of light, b) waves localized, standing, vortex. And these kinds of solutions are not exhausted. It is very likely that some kinds of localized solutions can also propagate to infinity at the speed of light. And it is very likely that many waves propagating to infinity have a localized structure. All these kinds of oscillations really exist in the universe, creating a visible variety of material objects.
More later. There is an assumption that all material objects existing in our perception are localized. Including radio waves.
Definition. One of the solutions of equation (1-1) is a localized wave. This is a vortex-shaped wave object localized in space - the field of stresses in Gukuum. The basic solution of the wave equation, which is used in the theory of gukuum to describe localized waves, is the sinusoidal spherical standing waves.
We work in spherical coordinates:

x = r•sinθ•cosφ, y = r•sinθ• sinφ, z = r•cosφ ;

A particular solution of the wave equation, spherical standing waves:

(1-2)

Where J_j+1/2 is a spherical Bessel function (simply) or, which is the same, a cylindrical Bessel function of the first kind:

     Y_j(θ,φ) - spherical surface harmonics;
    Y_j(θ,φ)=Ф_m(φ)•P_jm(cosθ) ;
   Y_j(θ,φ)=P_jm(cosθ)•Ф_m(φ)= P_jm(cosθ)•(a_mcosmφ+b_msinmφ) ;
   P_jm - an associated Legendre function of type 1, of order m, and rank j:

(1-3)

Ф_m(φ)=(const₁•cosmφ+const₂•sinmφ) ;

Further in the formulas, the quantity k , the figures are given in the pictures k=1/λ . On subsequent pages it will be shown that this value for different particles is different and equal to:

   It is suggested that the velocity of motion of a perturbation in a localized wave is equal to the velocity of transverse waves or the speed of light.
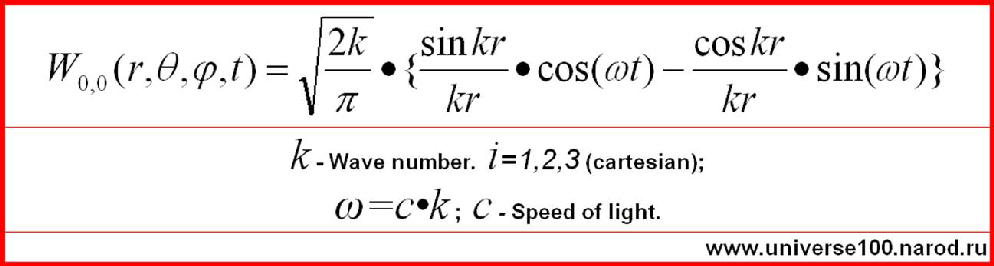
   In localized oscillations corresponding to the solution (1-2), at first glance, there is not only a circular oscillation, there is no transfer of energy at all. These are truly standing, vibrating vibrations in one place. But that's the situation in fact.
   CLASS 1. Allegedly localized "radiation sources" (traditional). The simplest case: j = 0 . Since equation (1-2) is linear, any linear combination of solutions (1-2) will also be a solution of (1-1). From (1-2), taking into account the fact that j = 0,±1, ±2,... It is possible to construct such a linear combination of solutions:

(1-4)

Whence it turns out, for j = 0 this is such a localized (!) Spherical wave:

(1-5)

It is difficult not to recognize in this solution the radiation of a point source. Physicists know that there is a flow of energy here! This is a mathematical trick.
An analogous linear combination for j = 1 also yields the dipole radiation known in physics:

And such combinations, probably, it is a lot of. For other j, the transformations are more cumbersome, and apparently multi-petal waves are produced. What objects correspond to these waves is a separate topic.
As will be shown below, this class of solutions actually determines localized wave objects moving with light speed. And specifically: photons and neutrinos. And other, not yet known in science education, moving with the speed of light.
The general formula for objects moving at the speed of light (photons, neutrinos and others):

(1-6)

True, a preliminary check shows that formally the energy integral over the given formula does not converge. But as we have seen before, you can not simply formally integrate. Necessarily somewhere there will be "winding", which must be taken into account. Maybe this test should be done better. The following reasoning may apply. The fact is that photons - they are here, are formed before our very eyes, in the present tense and massively. Therefore, at the time of formation, their shape is very far from the formula described above. Then, during the flight, they gradually relax to a normal form and all this happens at light speed. That is, the photon is already in the process of flying gradually grows this "divergent as an integral tail." This tail, despite the fundamental infinity of its energy in infinite time, remains at any finite time not too large in percentage to the energy of the photon center. But they always remain with the finite, initially given energy. By the way, is not this the cause of the cosmic "red shift" ?!
CLASS 2. You can try to apply the above focus not to the variable r, but to the variable φ. For example, the solution (1-1) can be the following linear combination of solutions (1-2):

(1-7)

Or after an obvious transformation:

(1-8)

And this is not the radiation of the point source, but the perturbation running around in a circle.
   Even at m = 0, the energy is still moving. The thing is that the gukuum itself does not move, it vibrates on the spot. Like a flickering chain of people on the fire, passing buckets of water to each other. But the energy of this vibration moves, like buckets of water. Sometimes two counterflows of energy create the appearance of neutralization of each other, and then a solution (1-2) is obtained.
   We call solutions (1-8) solenoidal. In such localized oscillations, energy moves around an axis.
   For simple solutions with m = 0, there is also an axial symmetry.
  As will be shown below, this class of solutions actually defines "inactive" localized wave objects. Specifically: all the elementary particles known to us, the proton, the neutron, the electron, the mesons, and so on. And other elementary particles, not yet known in science.
   CLASS 3. But the focus does not end there. How is the variable θ worse? There are adjoint functions (see solution (1-2), (1-3)), which can be represented in the form of products:

P_j,m = P*_j,m•sinθ ; и P_j,m=P**_j,m•cosθ ; (1-9)

for example,

P_2,1 = - 3•sinθcosθ; P*_2,1 = - 3•cosθ ; P**_2,1 = - 3•sinθ ;

To these solutions, you can apply the focus described above, not only to the variable φ, but to the variable θ. Here, it is not the aim of a complete investigation of all possible solutions of the wave equation. But experience tells us that it is also possible to carry out a similar linear combination of solutions (1 - 2) with respect to θ and get something like:

(1-10)

   In such objects, the energy does not rotate around the axis, but around the imaginary toroidal core, with the entrance inward. We call such localized oscillations toroidal. Their research is also a separate issue. It seems that in toroidal coordinates this will be simpler, more beautiful and there will be no singularities.
   I remember the ball lightning. Here is its field (not purely electromagnetic!), Rolled up with a lightning (this process is supposed to be by us in the formation of ball lightning, see below) as a fingertip from a finger (or according to Lermontov, as a thimble) is exactly toroidal.
   So, here is the hypothetical formula of ball lightning (naturally, in spherical coordinates):

(1-11)

CLASS 4 (optional). A similar situation with a flash lightning. Only there the field does not roll down, but remains after the slow lightening of the lightning. A solenoidal field is obtained, only in cylindrical coordinates. So the mathematical focus gets practical realization. There is a certainty that there will be many such mathematical tricks.
We work in cylindrical coordinates:

x = ρ•cosθ, y = ρ•sinθ, z = z ;

The main solution, which has a physical meaning, has the form:

(1-12)

Here i=1,2,3 (Cartesian); m - Integer; c_m, γ, k, K - Arbitrary; w=c /λ ; c - Speed of light. Z - Arbitrary cylindrical Bessel functions. But a continuous solution gives only Bessel functions of the first kind. These are sinusoidal cylindrical waves.
This solution mathematically should be a kind of endless garland sausages along the axis Z . And if it is physically feasible, then it is very likely that this object will turn out to be an Anniversary Lightning. Some analysis of this decision is made, here it is not given. The energy integrals converge (in terms of one sausage). But we postpone its presentation for the future.
In addition to the cylindrical solution, one can certainly carry out work as well as over a spherical solution. That is, similarly to find those three types of solutions, and the corresponding objects that generate the solution of the wave equation in cylindrical coordinates.

Опубликовано: https://www.academia.edu/34414309/Three_classes_of_localized_spherical_solutions_and_one_class_of_cylindrical_solutions

http://vixra.org/abs/1801.0240

Назад Главная страница Оглавление Далее

Страница размещена на сайте в мае 2005 года