внутреннее устройство элементарных частиц, портреты элементарных частиц, таблица элементарных частиц, размеры элементарных частиц, the essence of universe, the nature of matter, the sizes of elementary particles

Назад Главная страница Оглавление Далее

Lok dimensions.

Abstract. Estimates of the sizes of loks, assumed elementary particles are made. A complete equality is established between the effective dimensions of the loci and the Compton dimensions of the elementary particles.

   The basic solutions for loks contain indefinite constants, to which no special attention has been paid so far. Now it's time to clarify these vague constants, express them through world constants, and write out exact formulas for elementary particles.
   Thus, the basic and general formulas for all loks ([22] - [25]). These are formulas obtained on the basis of numerical experiments on many loks.
   The energy of the lok (j,m).

(1-49)

The angular momentum (spin) of the lok (j,m).

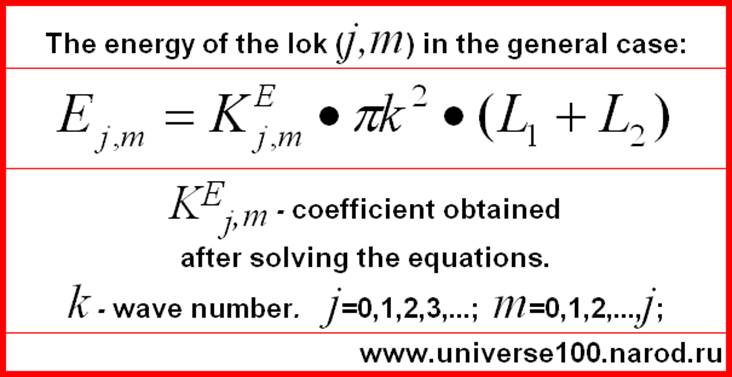
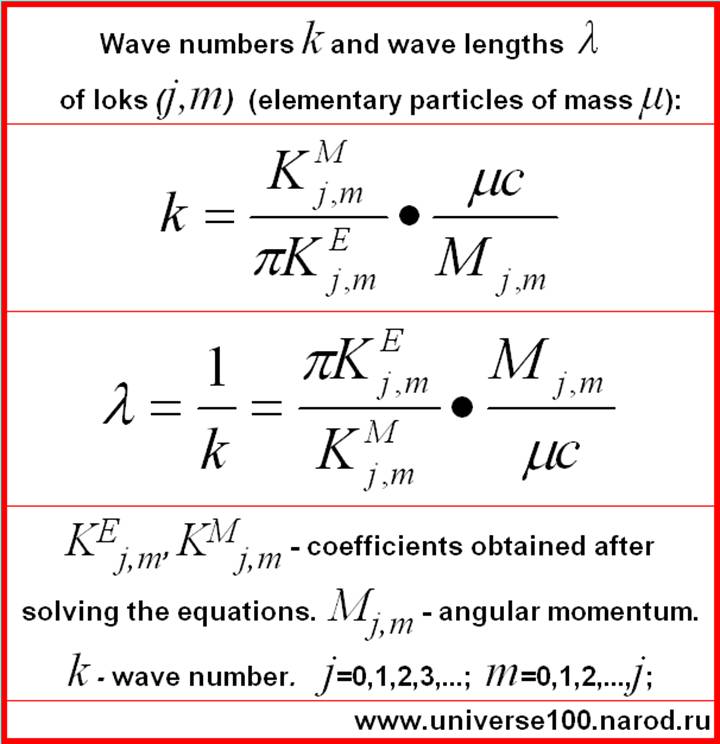
(1-50)

Thus, for each loks (j,m) we obtain a system of two equations with two unknowns k and (L₁+L₂). In the left-hand side of equation (1-49), the known experimental values of μc² must be substituted for each putative particle. The known experimental spin M_j,_m of each particle must be substituted into the left-hand side of equation (1-50). By dividing one equation into another, we get:

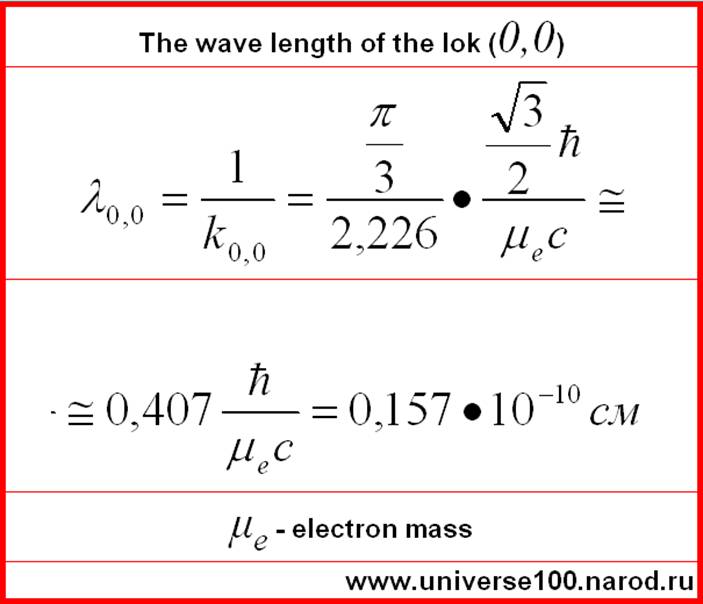
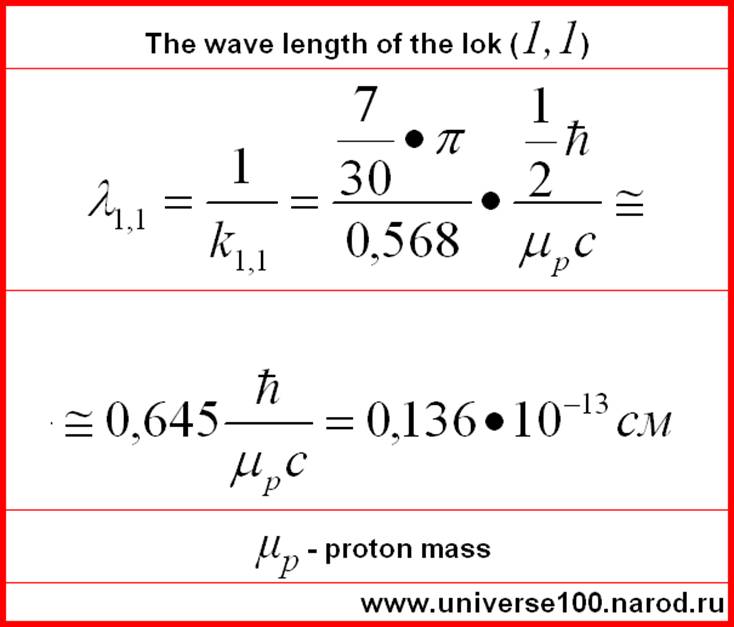
(1-51)

Whence are some wave lengths λ of the assumed elementary particles. They are equal to one division on the q axis in the graphs of Figures 4,5,7. Calculations are made immediately to the numbers, this will be needed. In accordance with formulas (1-23) and (1-42):

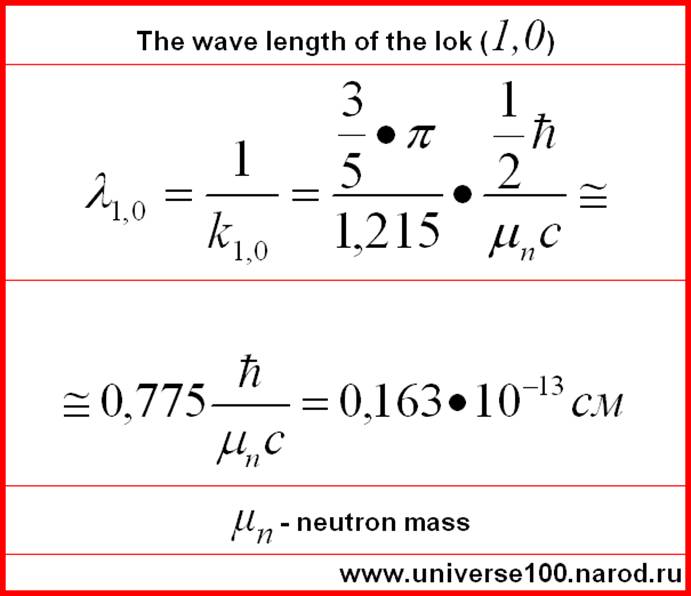
(1-52)

In accordance with formulas (1-26) and (1-44):

(1-53)

In accordance with formulas (1-32) and (1-46), an intermediate result is achieved:

(1-54)

The formula (1-48) is recalled and the reasoning related to it is that in the formulas (1-46) and (1-54) instead of the coefficient of 0.568, because of the non-sphericity of the lok (1,1) there must be a number of 0,76. Therefore, we can consider not sinning against the truth:

(1-55)

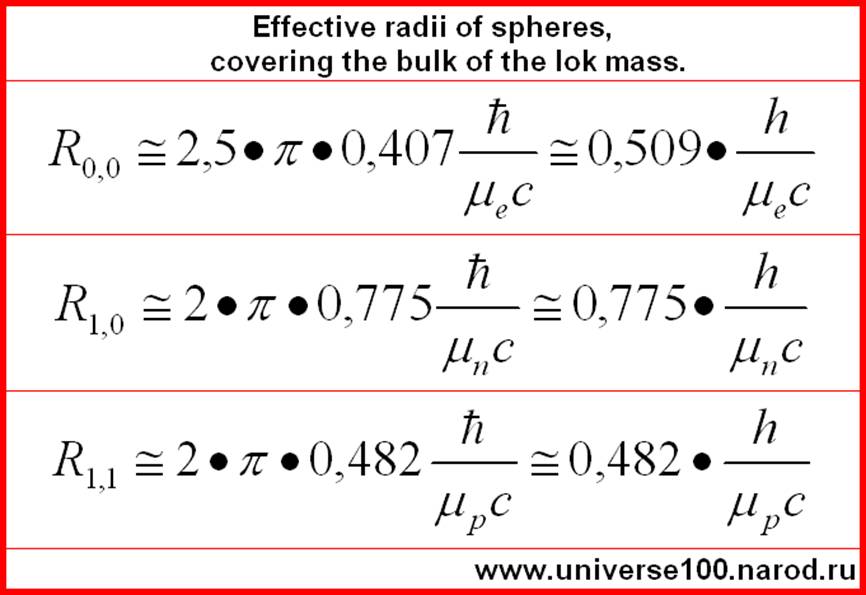
What relation have the values, λ_0,0, λ_1,0, λ_1,1 to the sizes of loks? If we look at the previous graphs of the distribution of the density of loks, we see that the masses of loks are distributed wavy, with decreasing. The effective radius of each lok, up to the radius covering the main part of the mass (Figure 4.5.7, per eye) is approximately equal to:
R_0,0≈2.5•π units of q; R_1,0≈2•π units of q; R_1,1≈2•π units of q.
In accordance with the formulas (1-52), (1-53), (1-55) we obtain:

(1-56)

Where h is the usual Planck constant not crossed out.
Having eyes, let's see: the effective radii of the loks (0,0), (1,0) and (1,1) are almost exactly the Compton radii of the electron, neutron and proton.
Still it is necessary to specify all results. But the moment of triumph has come.
1) The closeness of the proportionality coefficients in (1-56) for all three loks is absolutely obvious and natural. But could it be otherwise? Because the nature of all matter is one. In what theory is the size of the particles inversely proportional to (1-56) their masses ?! In what theory are the proportionality coefficients for the different loks (elementary particles) (1-52), (1-53), (1-55) and (1-56) practically the same? In which mathematical model, by substituting in the formulas the values of the particle masses, one can obtain theoretical particle sizes equal to their Compton (1-56) lengths?

2) Electron, as expected, is slightly smaller in size than in 1900 times each. In what theory is the relationship of particle size and mass exactly the same as the ratio of sizes and masses of real particles ?! This unambiguously proves that the form of elementary particles is exactly such.
   Proof number 3. The coincidence of the sizes and masses of candidate loks for identification with the ratio of sizes and masses of real particles is the third proof of the correctness of the theory of gukuum.
   So far, the values of C_j,m from (1-49) and (1-50) are unknown. They are expressed only through the parameters of the gukuum (L₁ and L₂). But, apparently, they were not needed. Because the solution (1-52), (1-53), (1-55) can not be considered simply "successful". This luck obviously consists in the fact that the coefficients C_j,m must initially have been taken to be identical and included in (L₁+L₂). Let the specialists - mathematicians decide.
    There is an interesting circumstance connected with the analysis of the linear dimensions of a free electron and a hydrogen atom, that is, in essence - an electron bound. It turns out that a free electron, having dimensions according to our theory, (1-56) equal to Compton (R_cmpt=1.21•10^{-10
cm) turns out to be about 40 times smaller than the size of
the hydrogen atom (the first Bohr radius is equal to:}R_bohr=0.53•10^{-8
cm.). There is nothing to be surprised at. When hydrogen is
formed, the electron changes its shape and expands. At the
same time, it still envelops the proton. The assumption that
the electron does not change its shape during proton
formation and revolves around the proton is rejected by us.
Initially, the proton is 1900 times smaller than the
electron and easily climbs into the central hole of the
electron. And then, when the electron interacts with the
proton, the electron is inflated 40 times more, which is not
surprising, since we do not yet know how the layers of the
electron interact with the proton layers.}

Опубликовано: https://www.academia.edu/34538843/Lok_dimensions

Назад Главная страница Оглавление Далее

Страница размещена на сайте в мае 2005 года