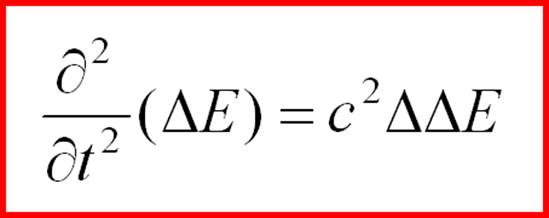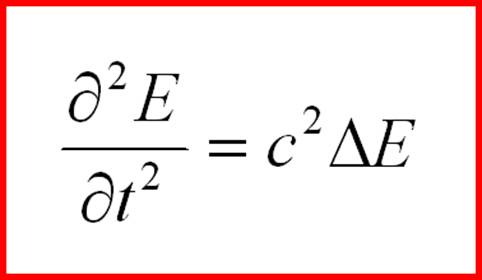|
Назад
Главная страница
Оглавление
Далее
The emergence of
quantum mechanics in a gukuum. Part 1.
Abstract. The identity of the
wave equation and the quantum Schrödinger equation is shown.
The exact physical meaning of the Heisenberg uncertainty
principle is obtained. The exact physical meaning of the
diffraction phenomenon is obtained. The exact physical
meaning of Planck's formula for the quantum energy is
obtained. An estimate of the dimensions of the lok is made
and its closeness to the Compton size of the elementary
particle is established.
Identity of the Schrödinger equation with wave equation.
In the first part of the undertaken description of the
universe, the main thesis was formulated: The universe is
homogeneous and elastic. All that is in the universe are
different kinds of vibrations of the universal Gukuum. The
elastic universe continuum is called a gukuum. The whole
universe is the Infinite Homogeneous Gukuum.
This model is not obvious and not natural for perception.
But she, like no other, absolutely blended into all the
known existing theories and simultaneously combined them.
So. All objects in the universe are described by the wave
equation:

(2-1)
W is the displacement vector of the
element of the elastic universal Gukuum.
Definition. We shall call the lok a localized vortex wave object, the
stress field in Gukuum. According to our theory of Gukuum, any
elementary particles are uniquely defined as Loks - localized wave
objects - stress fields in Gukuum.
It is interesting that the element of mass in the theory of Gukuum is
the element of stress in the Gukuum that moves at the speed of light.
Where and
mc2.
Now there is no need for duality, dualism. Material particles are
understood not as "point", without an internal device, but as real
spatial objects, with an internal device. Particles are not perceived as
"particles exhibiting some wave properties," but as real wave objects.
Even the particles actually do not exist at all, and there are only
Baki. The particles are not imagined as "smeared" in quotation marks,
that is, in the sense of
Ψ-functions,
but as really smeared and also rotating with the linear velocity of the
element equal to the light one.
Despite the fact that the main clot of these loks is localized in a
small volume, the peripheral part of them is smeared quite far in space,
albeit with a rapid decrease in density. From this, the phenomenon of
diffraction of a beam of elementary particles, interference and many
other phenomena turn out to be quite expected. All (!) Phenomena and
paradoxes from microphysics receive a simple explanation.
Example: diffraction. Ambiguously folds for the lok span
through the slot. Can slip well, straight. And can be hooked at the bar
and drove into the side ... Depending on many conditions, there is a
different diffraction pattern. In any case, this process becomes
understandable without the involvement of psi-factors.
We will set as our goal the gradual revision of all the provisions of
modern physics. Water stone hollow. Someday the quantity will go into
quality, the iceberg will melt away and
turn over.
The physical picture is always multifaceted. And that there were no
distortions when it is displayed, it is necessary to establish the ratio
of all its elements according to the principle "everyone with each." As
in the standings. It takes time.
Quantum mechanics arises extremely easily, clearly, naturally and
inevitably from the wave equation in Gukuum. No assumptions and strains
are needed. Even no experiments are needed - everything has already been
tested. Postulate Gukuum and deduce the theory of the whole universe.
The theory of Gukuum is none other than the Axiomatic Physics, the
necessity of which has been spoken for so long during the Bolsheviks.
It is even surprising how simple the words are to be made, so that
the cause of the discreteness of the universe becomes understandable,
the cause of quantum mechanics.
There are many solutions to the wave equation, but they can be
conditionally divided into groups that exist in the Gukuum themselves,
interact and exchange energy only within the group, and do not affect
the other groups in any way. One such group is a group of discrete
solutions, in which several integer parameters appear. This is our
universe. Regarding the fate of the other groups of decisions, we are
not very tense.
The discrete solutions obtained from the wave equation largely
coincide with the solutions obtained from the Schrödinger equation. A
complete analytical analysis of all quantum mechanics on the theory of
Gukuum will require a long effort. This is work for whole institutions.
This is a reworking of all textbooks.
Principle of uncertainty
The existing quantum physics
seems to offer more equations than the parameters of the
object under study. Hence the impossibility of simultaneous
measurement of some pairs of quantities, hence the principle
of uncertainty.
According to Gukuum theory, the uncertainty principle
arises somewhat differently. Banks do not have a strictly
defined form. They have nothing stable at all. These objects
are vibrational - rotational, with an internal
transformation of energy between kinetic and potential. Also
with frequent, directly-machine-gun, exchanges of thermal
and light quanta with surrounding particles. Any measurement
above the lok affects its state. Example: passing an object
through a slot. This experiment - fixing the width of the
object. It is similar to the processes that we observe in
everyday life when pushing something rounded into a slot,
for example a ball between the goalkeeper's legs or
swallowing a piece of cake. At the moment the object slips
through the slot, it first moves with difficulty, contracts
laterally, and stress is required to push it through the
slot. Then, after passing through the narrow part, it is
again unclenched and accelerated. As a result, it is at the
moment of measuring the width of the object that there is an
uncertainty in its momentum. This uncertainty sometimes ends
sadly. Or an unexpected goal "in the point" goalkeeper or
vice versa, stuck in the throat or esophagus piece of cake
(or worse - in the evening constipation). And few people
suspect that the principle that was discovered by Heisenberg
is to blame.
Analogous results also appear in the measurement of other
parameters in loks. For example, in an energy measurement
experiment, the longer the measurement time, the better the
measured energy is averaged, its scatter is smaller and its
mean value is measured more accurately. Conversely, the
shorter the measurement time, the more uncertain the energy
data, because it jumps back and forth from the kinetic to
the potential, from one "petal" object to another, not to
mention the exchange of energy with other particles.
In any experiment to measure a
parameter, the lok is fixed by the same parameter and is
limited. As a result, some other parameter is changed. This
is an ancient law, coming from Lomonosov.
Conclusions. A clear (not formal-mathematical)
explanation of the Heisenberg uncertainty principle, which
has no such explanation in quantum mechanics, is a small
first signal in favor of the theory of Gukuum.
The
Schrodinger equation.
It is quite obvious that the Schrodinger equation and
the wave equation in the elastic body are similar in
relation to the theory of Gukuum.
According to the textbook, we have the Schrodinger
equation for a moving particle:
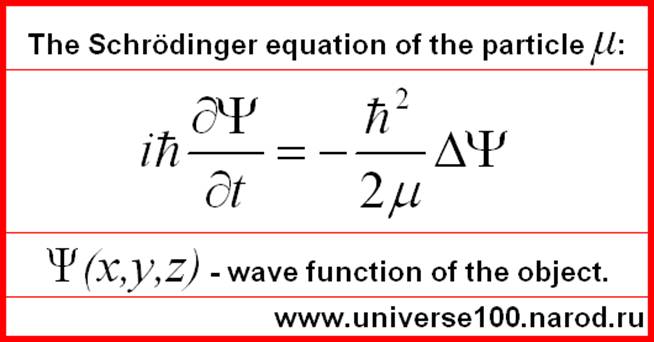
(2-2)
Here
Ψ=Ψ(x,y,z)
is the wave function of the object. The square of this function, in
traditional transcription, has the physical meaning of the probability
density of finding a "particle" in an element of space. What kind of
"particle" will it be discussed below. Further,
ћ
is the Planck constant,
μ
is the rest mass of the particle.
Note. For uniformity, the "crossed" Planck constant and all the
formulas and quantities associated with it are taken everywhere.
The Schrodinger equation is transformed into the wave equation and
vice versa:

(2-8)
where
Wx
is the
x-component
of the displacement vector
W
of the elastic medium element. It is not difficult to see
that the physical meaning of
Wx
is close to the physical meaning of the above-mentioned
Ψ(x,y,z).
Namely, the square of the displacement of the element of
Gukuum carries in itself the meaning of the element of the
stress energy (Hooke's law), and consequently the element of
the mass of the object. Consequently, the square of the
displacement
Wx
also has the meaning of the probability of finding a
particle of mass in an element of space. As will become
evident from the following, this closeness is of the nature
of complete coincidence.
The formula obtained below will serve to obtain two more
interesting formulas. First we obtain a formula for the
energy of the lok, analogous to Planck's formula:
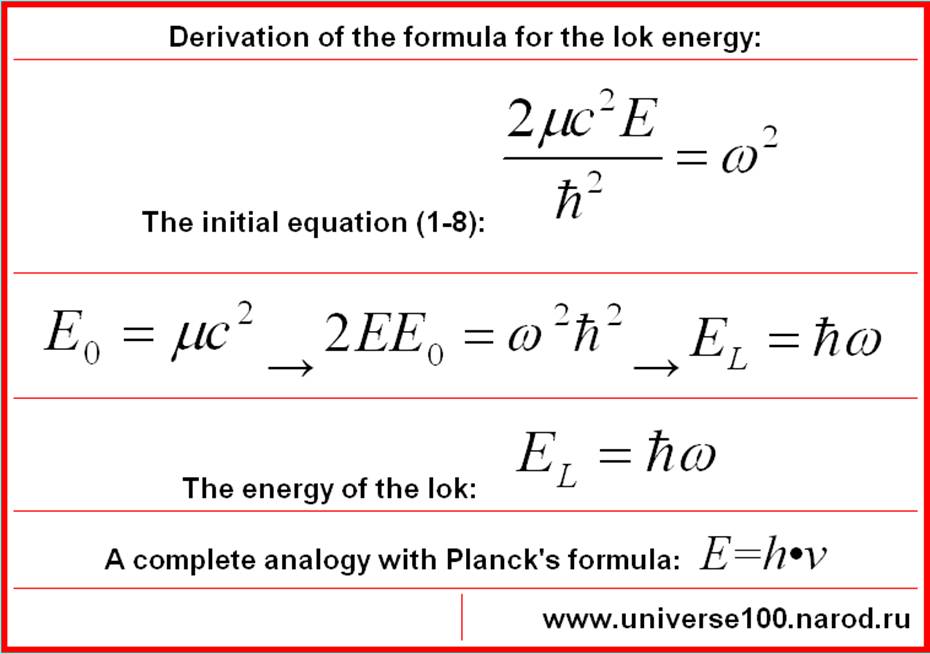
Here,
E0 has the meaning
of the total energy of the particle. For a lok, the value of
E initially had the meaning of
kinetic energy. But since this object is not pointlike, but
complex, according to Lomonosov's law of equilibrium, it has
the same amount of potential energy. The total energy of the
lok is
EL = 2E. And it is
equal to
E0.
The
value of
ω,
as mentioned, has so far a certain supposed average meaning,
because different parts of the lok, in principle, can rotate
at different angular velocities. Below we will estimate this
angular velocity of rotation.
If the obvious formal substitution is made in equation
(2-8):
ω=2πc/λK,
again to take into account the Kelvin formula and find this
λK,
we get the familiar expression:
Then, from the same basic formula, we obtain a formula
analogous to the Compton wavelength:
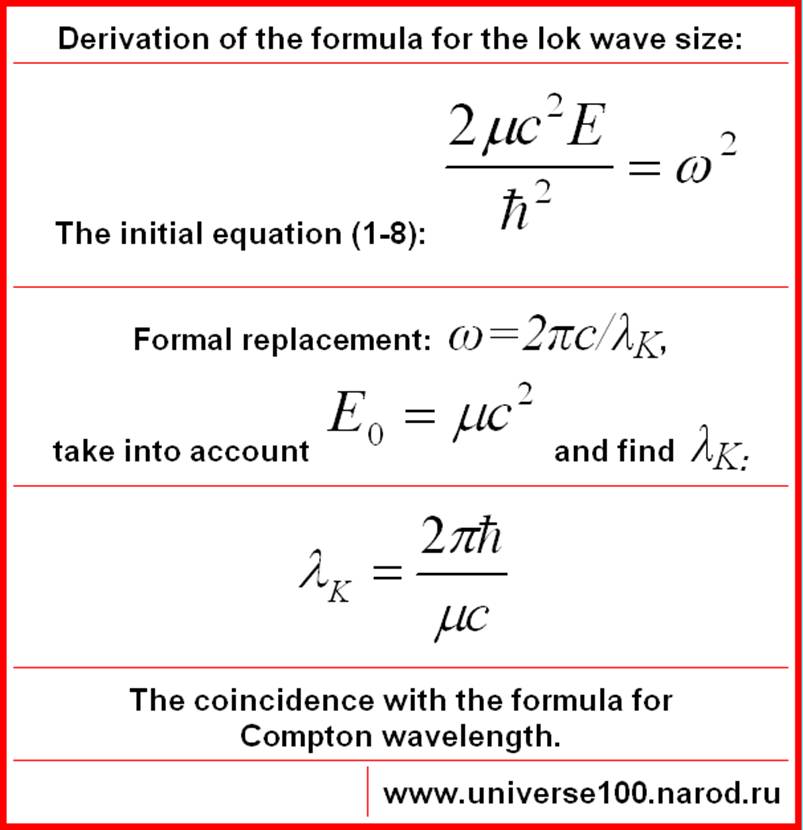
It
is not difficult to determine the Compton wavelength in this
quantity and formula.
Conclusions. The above proof of the identity of the
Schrödinger equation and the wave equation is unlikely to
say anything new to mathematicians. However, it slightly
lifts the veil over the mystery of the mathematical
apparatus of quantum mechanics. It is of interest to
physicists. And this is the second signal in favor of the
theory of Gukuum.
On the sense of linearity of quantum operators.
Well, the offset in the Gukuum correlates with the wave
function by 100%. But this does not mean that all the
remaining quantum physics built on linear operators will
also be well correlated with the wave equation. For example,
how does the wave energy in Gukuum behave ?!
Having done all the necessary calculations, we conclude
that:
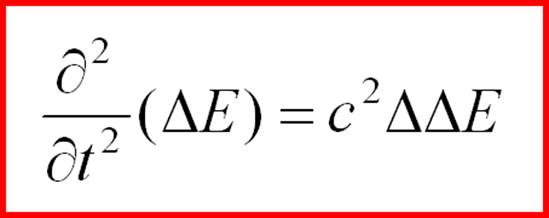
(2-21)
What
generally does not mean that the energy in the gukuum also satisfies the
wave equation.
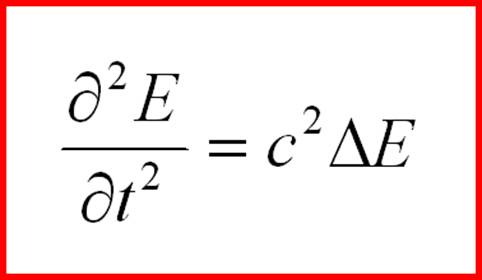
(2-22)
However,
for many problems this is the case. Here we need further analysis, which
we are putting off for now.
If there is time, similar calculations will be done with all possible
physical quantities (momentum, momentum, etc.) for the loks. So far, a
reasonable assumption has been made that some of them satisfy the wave
equation. In particular, the impulse.
Thus, the artificiality of the introduction of operators begins to be
revealed, as is the Schrödinger equation and all of the current quantum
physics. However, this is not a secret. The introduction of operators is
dictated by their convenience, linearity, which, as we saw, stems from
the wave equation. The introduction of operators is dictated by the
complexity of the problem. What kind of task? And the only task of
understanding reality - Gukuum. And this is the third signal in favor of
the theory of Gukuum.
All relatively quantum physics, which affirms the absence of the
chosen frame of reference, is built just on the chosen frame of
reference: the count from the person, from his perception. We are firm,
and there is emptiness around. An, it's not so and vice versa! There is
no doubt that it was worthwhile to tell someone about 60 years ago about
Gukuum, but to insist on it, so that physicists believe, then it would
be "a matter of technique" to create a mathematical apparatus. The whole
complexity of the unusual, and therefore Einstein rejected this version.
Yes, the equations of propagation of electromagnetic waves, light are
similar to the equations of sound waves in an elastic medium. "But what
is this environment in which light is spreading and what are its
mechanical properties?" While this question remains unanswered, there is
no hope of reducing optical phenomena to mechanical ones, but the
difficulties in solving this problem are so great that we must abandon
this path , therefore, they must also abandon mechanistic views
"(Einstein, citation, [2]). And all of his followers, like his
predecessors, did not even try to give meaning to this idea.
Continuation
of the chapter on the next page.
*
Опубликовано: https://www.academia.edu/34572741/The_emergence_of_quantum_mechanics_in_a_gukuum._Part_1
Назад
Главная страница
Оглавление
Далее Страница размещена на
сайте в мае 2005 года |